3D Or Three-Axis Calibration: What’s The Difference?
Guarding against errors in the volumetric positioning of a machine tool is critical to machining contours and other 3D surfaces accurately.
Many shop people think three-axis accuracy and 3D accuracy are the same. The truth is that three-axis accuracy is one-dimensional because it specifies only the tolerance of linear measurements along each axis. 3D accuracy refers to linear measurement of each axis and the relationship of the X, Y and Z axes to one another—that is, the straightness and squareness of each axis to one another within a defined work cube.
Calibrating three-axis accuracy is relatively simple and is useful for identifying such problems as leadscrew/ballscrew pitch error or wear. Calibrating 3D accuracy is more complicated but doesn’t necessarily take more time. However, it is a much better way to ensure the overall performance of a machine when cutting contoured surfaces and other 3D parts designed with 3D CAD software. For any shop, knowing when and how to do these different calibrations is important because each provides different information about machine performance.
Before launching into the differences between three-axis and 3D calibration, it’s helpful to understand that most machine tool positioning systems are based on the Cartesian coordinate system, which uses a series of points along three coordinate axes (X, Y and Z) aligned perpendicular to one another to represent 3D objects or features.
Much of the confusion surrounding three-axis and 3D calibration has to do with terminology. A shop that just calibrates linear displacement along each of the three axes may consider this three-axis calibration. However, the three axes are not calibrated for 3D accuracy because linear displacement does not consider the perpendicularity of the axes to one another.
Based on rigid body geometry, which defines positions by forming 90-degree angles with an axis of a given reference frame, each of a given machine tool’s three axes is susceptible to six errors for a total of 18. These six include three linear errors as well as pitch, yaw and roll angular errors, respectively. Taking into account three potential squareness errors leads to a grand total of 21 possible rigid body errors for a three-axis machine tool. By calibrating linear displacement error along each axis, only three errors will have been determined, leaving 18 errors undetermined.
Three-Axis Linear Calibration
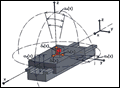
Linear displacement along an axis of a CNC machine can be calibrated using a system based on laser Doppler displacement meter (LDDM) technology. This requires only two optic elements, which are temporarily mounted on a machine tool or coordinate measuring machine. This makes setting up the system and aligning the beam relatively easy and quick. The laser in this application meets standardized traceability requirements and features a stability check of better than 0.1 ppm, accuracy of 1.0 ppm and resolution up to 1 microinch.The laser reading head is mounted on the bed or table and a retroreflector (also called a target) is mounted on the spindle. The tuned laser beam aligns parallel to the axis. The operator programs the measurement increments along the axis. The spindle with the retroreflector starts at the home position. The system then moves the retroreflector to each specified incremental position and records the measurement. Incremental positioning and data capture can be accomplished automatically or manually.
This process identifies deviations by comparing the measurement scale to the positions measured by the calibration system. These deviations are then used to calculate a compensation table. Some situations call for the application of a single linear correction factor. Others require incremental pitch correction factors—that is, errors may occur in only specific areas and are not uniform across the axis.
Relying on linear calibration (one-dimensional measurements parallel to the axis of movement) assumes that the only possible errors are leadscrew/ballscrew and thermal expansion errors. Linear calibration along three axes is inadequate for ensuring accuracy of 3D parts. Many years ago, national and international standards-making bodies recognized this and introduced the ASME B5.54 and ISO230-6 machine tool performance measurement standards.
3D Calibration
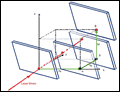
The ASME B5.54 and ISO230-6 standards resulted in two methods for 3D (volumetric) calibration, including the “body diagonal displacement method” and the proprietary “sequential step diagonal measurement method.” For years, the body diagonal displacement method defined by ASME B5.54 and ISO 230-6 has provided a quick check of volumetric error with good results. Because the measurements involved are relatively simple and quick to make, the cost and machine downtime are minimal.The body diagonal displacement method is a measurement of the volumetric positioning accuracy of a machine tool with a laser calibration system. A laser is mounted on the machine bed, and a retroreflector mounted on the spindle reflects the laser beam, which is aligned along the machine diagonal.
With the laser pointing along the body diagonal direction and the retroreflector moving along the body diagonal at operator-specified increments, the laser calibration system records measurements at each position. Measuring the displacement error begins at the home position and at each increment along the three axes, which move together to reach a new position along the diagonal.
The last four body diagonals use the same corners as the first four diagonals, except the directions are reversed. For that reason, there are only four body diagonal directions with forward movement and reverse movement (bi-directional) and only four setups in which measurements are taken after each simultaneous move of X, Y and Z. The accuracy of each position along the body diagonal depends on the positioning accuracy of all three axes and geometrical errors of the machine tool.
In theory based on the calculation, the four body diagonal displacement errors are sensitive to all nine linear errors, which may be positive or negative; and these nine may cancel each other out. Because the errors are statistical in nature, the probability that all of the errors will be cancelled in all of the positions and in all four of the body diagonals is theoretically possible but highly unlikely.
However, the body diagonal displacement method does not clarify the relationships between the body diagonal displacement errors and the 21 possible rigid body errors. Another concern about this method is that it assigns too much importance to angular errors. To understand the relationships and importance of angular errors, it is necessary to derive the relations between the 21 rigid body errors and the measured body diagonal displacement errors.
Based on the above-derived relations, all the angular error terms are cancelled except for two. Therefore, the body diagonal displacement errors are sensitive to displacement errors, straightness errors and squareness errors but not angular errors. Because there are only four sets of data and nine sets of errors, the body diagonal displacement method does not generate enough information to determine the source of errors. Optodyne, a company that develops and markets laser calibration systems, developed the sequential step diagonal method to address these issues.
The basic concept of this method is that the laser beam direction (or the measurement direction) is not parallel to the motion of the linear axis. Therefore, the measured displacement errors are sensitive to errors that occur both parallel and perpendicular to the direction of the linear axis. More precisely, the measured linear errors are the vector sum of all errors projected to the direction of the laser beam, including the displacement errors (parallel to the linear axis) the vertical straightness errors (perpendicular to the linear axis) and the horizontal straightness errors (perpendicular to the linear axis and the vertical straightness error direction).
Collecting data with the laser beam pointing in four body diagonal directions identifies all 12 types of errors. Because the errors of each axis of motion are vectors with three perpendicular error components, this is considered a vector measurement technique.
During conventional body diagonal measurement, the laser beam moves along a body diagonal and collects data at each preset increment. During vector measurement, all three axes move in sequence along a body diagonal, and data is collected after each axis is moved. This method collects three times more data than the conventional body diagonal measurement, and it can separate errors according to the movement of each axis.
The sequential step diagonal measurement method differs from the body diagonal displacement measurement by moving each axis separately and in sequence, collecting the diagonal positioning error after each separate movement of the X, Y and Z axes. The trajectory of the target is not a straight line, and the lateral movement is quite large. Thus, a flat mirror must be used as a target.
The conventional linear displacement technique measures along only one edge and fails to consider pitch, yaw and angular errors. The sequential step technique measures along all four edges. The measurements are averaged to obtain displacement error through the center of the volume, which is believed to be inherently more accurate.
For example, pitch, yaw and roll angular errors affect all the measurements, including the linear displacement measured by a conventional laser interferometer. Therefore, the linear displacement errors measured along the X axis will be different when measured at different Y locations and Z locations. This is a result of different Abbé offsets at different locations and the pitch, yaw and roll angular motions. For this reason, the B5.54 standard states that all linear displacement measurements must be along three orthogonal lines, that is, parallel to the three axes, and passing through the center of the working volume.
The advantage of the sequential step diagonal measurement technique is that positioning errors caused by angular errors are measured and expressed as the averaged straightness errors along the center lines of the working volume. Because most machine tools cannot compensate for angular errors, this advantage is crucial. When angular errors can’t be compensated, the accepted work-around is to compensate for the averaged straightness errors. Note that the displacement errors and straightness errors measured along one edge of the working volume will differ from those measured along another edge because of the Abbé offset and angular errors. For this reason, the sequential step diagonal measurement technique measures and averages over the four edges.
The Growing Value Of 3D Calibration
Every shop has accuracy requirements peculiar to its customers, processes and machine tools. As 3D CAD/CAM systems are used to design more parts, the importance of ensuring that machine tools are capable of machining 3D parts accurately is growing. Because three-axis linear calibration does not consider the 3D relationships between each of the axis, only 3D (volumetric) calibration is acceptable for ensuring that a machine tool is capable of making 3D parts accurately.Regular 3D calibration and compensation leads to shorter cycle times, better-quality parts, less-frequent repairs and lower warranty costs. With vendor quality-control programs requiring calibration, the ability to utilize volumetric calibration and compensation will inevitably lead to more competitive and profitable manufacturing processes.
About the author: Charles Wang is the president of Optodyne Inc. in Compton, California.
Read Next
Keeping Accuracy Within Reach
Part quality is at risk if a machining center cannot hold tolerances at the farthest reaches of its work envelope. This makes volumetric accuracy a key indicator of a machine's performance. One machine tool builder discusses the implications.
Read More3 Mistakes That Cause CNC Programs to Fail
Despite enhancements to manufacturing technology, there are still issues today that can cause programs to fail. These failures can cause lost time, scrapped parts, damaged machines and even injured operators.
Read More













.png;maxWidth=300;quality=90)







